Invisible Handshake

On Feb. 20, 2008, a large stone sculpture by Helaman Ferguson was installed on the south deck of the Olin-Rice Science Center at Macalester College.

The Title

The title, officially Invisible Handshake II, refers to the fact that the shape is topologically equivalent to the space between two hands that come together to grasp each other in a traditional handshake, but stop just short of touching. There are four large passages, in two pairs; they correspond to where the thumb and fingers of each hand would go. In the image below, one hand would go into the orange upper bowl and come out below the central skirt, while the other does the symmetrical thing from the lower blue bowl, emerging above the skirt.

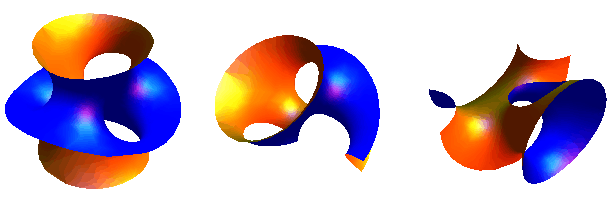
The following images of a model show the two Y-shapes.

Inspiration

The form arises from a shape discovered by Celso Costa of Brazil in 1984. That shape is related to energy-minimizing surfaces that arise naturally in the physics of soap bubbles; see photo of a soap bubble demonstration in sub-freezing conditions by Matthias Weber. Costa’s discovery marked the start of a new era in the investigation of these surfaces, technically called minimal surfaces.

This project in stone was inspired by a similar construction, Invisible Handshake I, created in 1999 at a snow sculpture competition.

Negative Curvature

This image shows positive curvature.

A surface has positive curvature at a point if the surface curves away from that point in the same direction relative to the tangent to the surface, regardless of the cutting plane. Alternatively, the surface stays on one side of the tangent plane at that point. Thus the top of your head, the end of your finger, or the inside of your armpit are points of positive curvature. The first image on the left, a bump, shows positive curvature.

A surface has negative curvature at a point if the surface curves away from the tangent plane in two different directions. The classic example is a saddle, which can be found on your body in the space between your thumb and forefinger, or along the inside of your neck. Any point on the inside of a torus has negative curvature because there are planar cuts that yield curves that bend in opposite directions with respect to the tangent plane at the point. Negative curvature — the saddle shape — arises spontaneously as nature tries to minimize energy; see the soap bubble photo above. Invisible Handshake is a form that has negative curvature at each point. Thus each point can be viewed as the keystone of two arches in different directions, and this feature gives the surface great strength.

Triply Punctured Torus

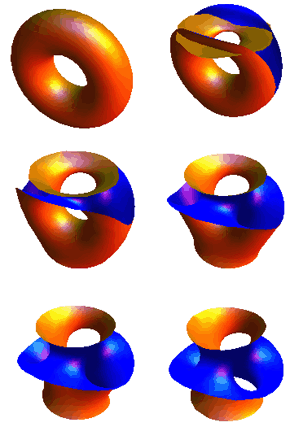
The sculpted shape is topologically equivalent to a torus with three punctures. The images, created in Mathematica using the late Al Gray’s parametrization of a Costa surface and simply morphing between that and a torus (an idea of Dan Schwalbe), show one can transform a triply punctured torus to a Costa surface.

The Formulas

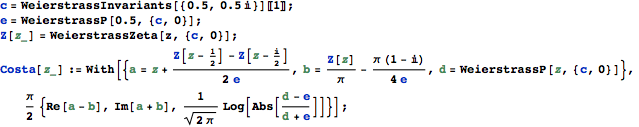
The formulas for the Costa surface are etched into the side of the sculpture, and involve complex numbers and special functions from the nineteenth century called Weierstrass elliptic and Weierstrass zeta functions. The Mathematica code below shows how one can define Costa in terms of these functions, where the domain is the unit square in the complex plane. That is, given InvisibleHandshake_10.gif with u and v between 0 and 1, one can define a point in 3-space by the Costa formula that follows. Because one can also go from a square to a torus, this provides the link between Costa and torus that is exploited to get the morphing movie in the Triply Punctured Torus section.

The Time Stamp

The time stamp on the sculpture beginning 2008022020384403141….. refers to the time of the lunar eclipse on the day of installation, in the Mountain Time zone (birthplace of the sculptor).

The Stone

The 11-ton block came from Raymond, California, via Cold Springs, Minnesota, and is commonly called Academy Black granite. The finished sculpture weighs 6212 pounds. This type of stone weighs about three times as much as water. The material is, in fact, not granite, but quartz diorite, and is 100 million years old. A geological analysis by Jeff Thole (Macalester geology department) showed that it is a medium-grained quartz diorite containing primarily plagioclase with lesser amphibole, orthopyroxene, quartz and biotite (in decreasing order of abundance). The image below is a thin section (30 microns thick) of the stone using cross-polarized light; the field of view is about one centimeter.

The Red Frame

The remnants of the sides of the original block form a frame for the sculpture and are painted red using a historically noteworthy dye called cochineal. This dye comes from Dactylopius coccusnative, a scale insect that is native to Mexico and feeds on red cactus berries. The insects produce a red-pigmented substance that is believed to serve as a defense against predators. The red pigment is harvested by cochineal “farmers”; it takes about 155 insects to make one gram of cochineal dye. The quality of the dye is strongly correlated with how much carminic acid it contains. Carminic acid, also known as natural red dye number 4, consists of anthraquinone, which is largely responsible for its red color, and glucose, a common sugar.